Bol Loops of Order 16 with 13 Involutions

As part of my enumeration of the Bol loops of order 16 with nontrivial centre, here I list just those which are non-associative with exactly thirteen involutions. Please see the parent page for notation, including my conventions for naming of loops and table entries. I would appreciate an email message () from you if you have any comments regarding this list.

All 117 loops in this list have |Z(L)|=2 and so the graph Comm(L) is defined. This graph is displayed as black-on-white if it has at most 10 edges; otherwise its complement (which has at most 10 edges) is displayed as white-on-black. In either case, isolated vertices are not displayed.

No.	Comm(L)	|C(L)|=2	|C(L)|=4	|C(L)|=6	|C(L)|=8
2		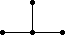			0
6			2
9		75
10		0
12		44
13		2
15		97
16		22
19		14
23		21
26		83
27		61
29		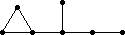17
32		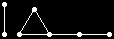91
33		1
35		81
40		59
44		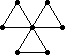6, 48
46		98
47		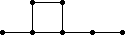40
51		78
52		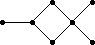80
55		103
57		64
58			7
60			10
63		36
65		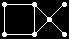26
67		63
69		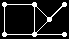56
71		38
73		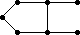8
76		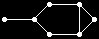42
78		92
81		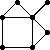3, 23, 79
85		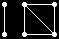82
86			1, 5
89		95
91		85
93		101
94		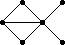	3
99		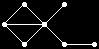52
101		60, 71
104		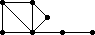31
106		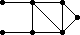72
108		84
110		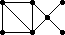47
113		89
114		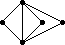		0
116		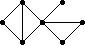69
118		13
121		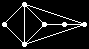46
123		15
126		39
128		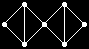99
130		66
131		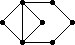41
135		32
137		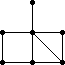93
140		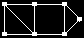43
142		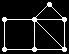86
144		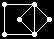94
145		4, 16
158		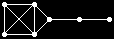74
160		65
162		73
164		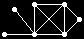12
166		35
168			0
170		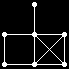68
173		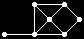54
175		18
176			6
180		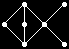102
181		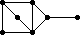29
183		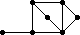62
186		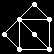45, 96
188		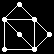19, 20
194		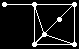77
196		37, 49
199		34
201		27
205		51
207		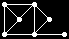50
209		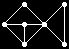55
211		67
213		11, 100
214		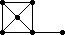	4
217		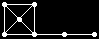76
220		53
221		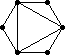	9
223		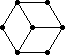5, 28
226		70
228		24
229		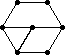30
231		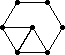25
234		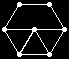9
236		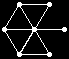	8
238		57
240		58
242		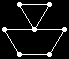87
245		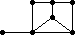7, 10
247		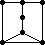88
250		33
252		90

/ revised November, 2001