

Instructional videos for Math 4520 as hosted on YouTube are linked in the table below. Recommendation: Some of these videos are face-paced. Pause frequently and repeat; or try playing at 0.75 times normal speed (a feature in YouTube).
|
Textbook
|
Title
|
Duration
|
Link
|
Description |
|
§
|
32:55 | We place the real numbers in the context of the zoo of important number systems. | ||
|
§
|
24:09 | Decimals are such a handy way to express real numbers... it's tempting to forget that the real number system is not defined using decimals. | ||
| § | Equivalence Relations | 30:52 | 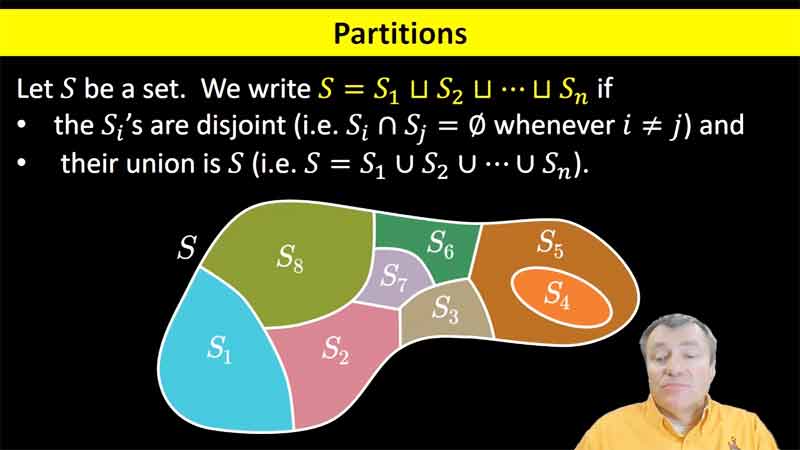 |
Equivalence relations serve as an extremely prolific tool for constructing new mathematical structures from old. |
| § | Complex Numbers | 15:27 | 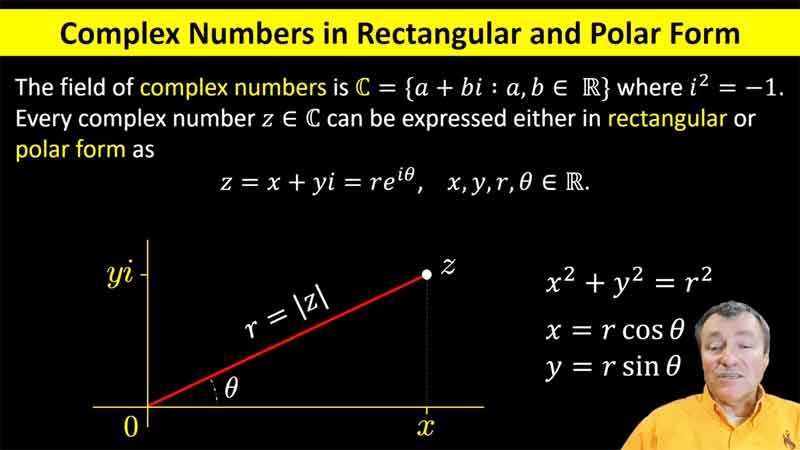 |
Much can be said about properties and applications of the complex numbers. Here we highlight some ways that the complex numbers shed light on the real numbers. |
| § | Polynomials and Series | 28:35 | 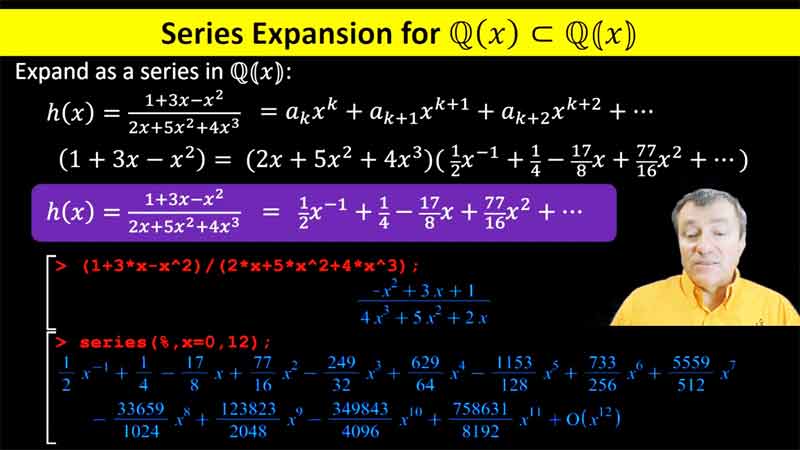 |
We introduce polynomials, rational functions, power series and Laurent series from a formal (i.e. symbolic) perspective. |
| Induction | 24:08 | 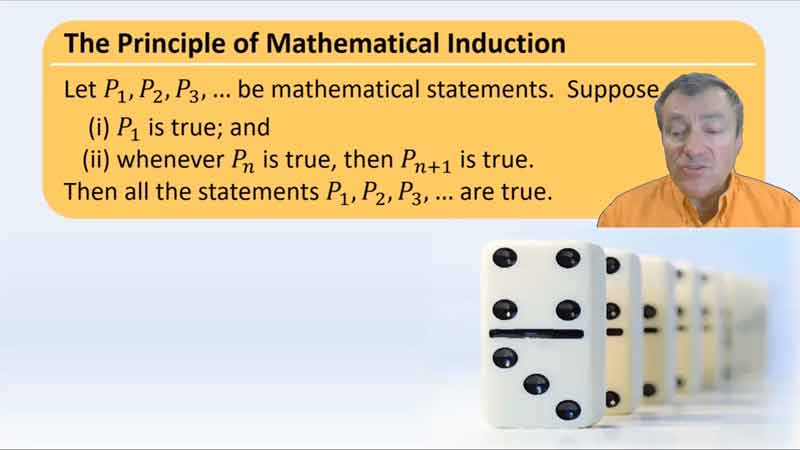 |
We introduce the Principle of Mathematical Induction, along with several examples. The Least Number Principle (the fact that the natural numbers are well ordered) is at the heart of induction. | |
| Interesting Numbers | 5:25 |  |
Which numbers are interesting? Are all numbers interesting? | |
| Normed Fields | 22:20 | 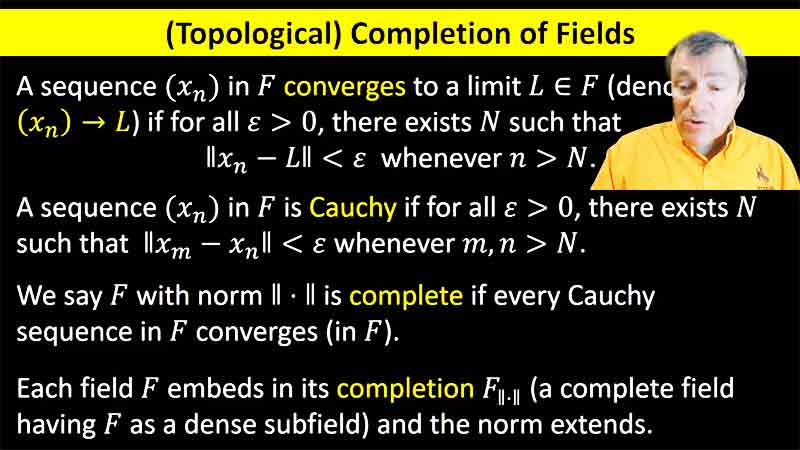 |
Several of our examples of fields (the reals, the field of Laurent series, and the p-adic fields) are constructed by the process of topological completion with respect to a norm. Here we discuss normed fields and the process of completion. | |
 / revised August, 2020
/ revised August, 2020